|
|
|
|
|
|
Let f be a continuous complex-valued function of a complex variable, and let C be a smooth curve in the complex plane parametrized by
We will consider line integrals of the following functions
over a varierty of different curves.
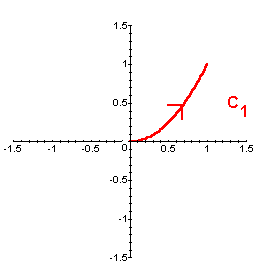
Repeat the calculation for the parametric representation
Now repeat the calculation using your own parametric representation for C1.
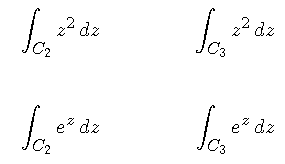
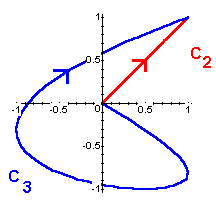
where C2 and C3 are the curves displayed below. Record your results. What do you observe about the values of the integrals?
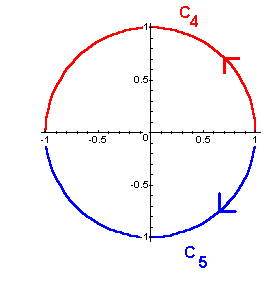
Calculate the integrals of all four functions over each of the two curves and record your results. (Check to make sure that your parametric representations trace out the curves in the correct directions. Also check the bounds on your parametric intervals.)
|
|
|
| modules at math.duke.edu | Copyright CCP and the author(s), 1998-2001 |